

All graphics and concepts presented in this note are also derived from the WWB Fourier transform utility.
FOURIER TRANSFORM SOFTWARE
But what should you look for in Fourier analysis software? What makes one software package better than another in terms of features, flexibility, and accuracy? This application note will present and explain some of the elements of such software packages in an attempt to remove the mystery surrounding this powerful analytical tool.įigure 1 - The Fourier transform illustratedĭATAQ Instruments' WinDaq Waveform Browser (WWB) playback software contains a Fourier transform algorithm that was the model for this application note and includes all elements of Fourier transformation discussed herein. Algorithms have been developed to link the personal computer and its ability to evaluate large quantities of numbers with the Fourier transform to provide a personal computer-based solution to the representation of waveform data in the frequency domain.
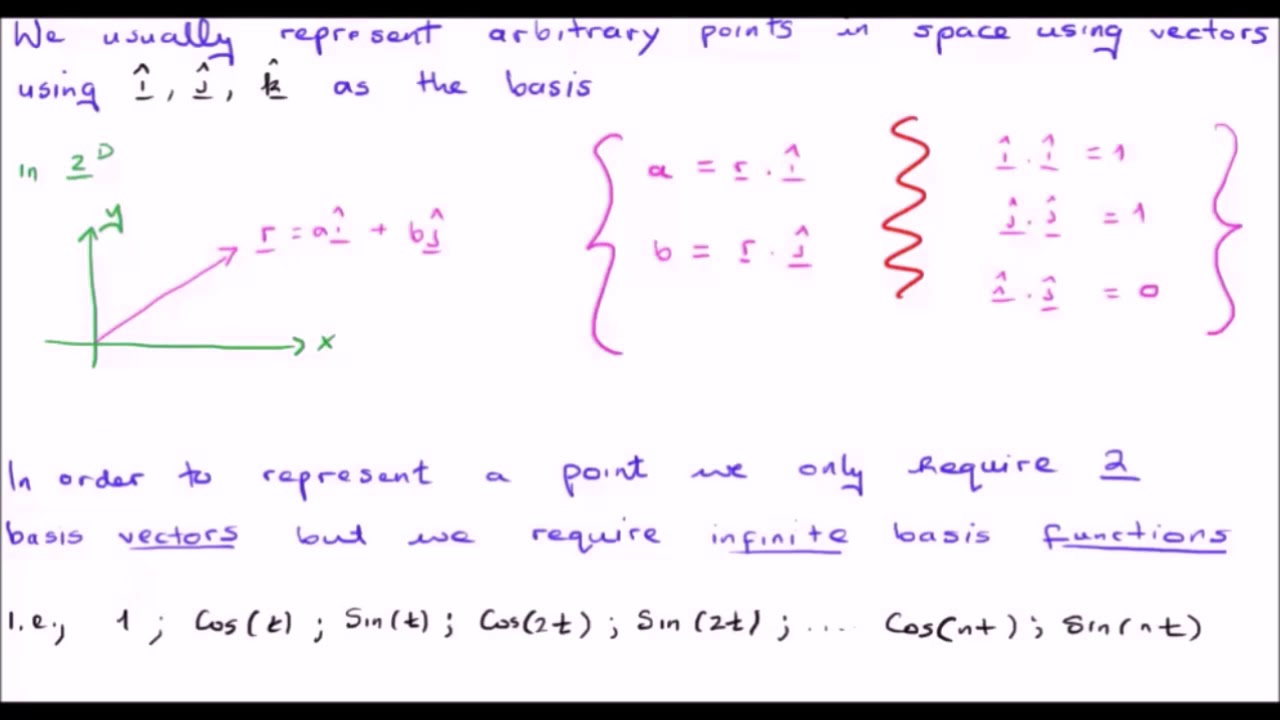
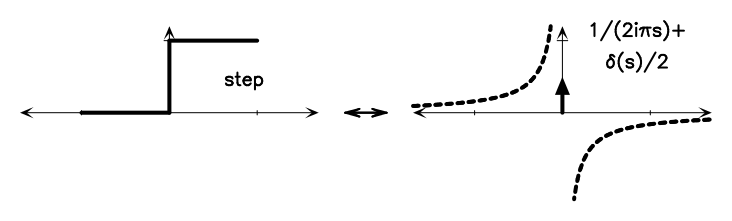
Perhaps because of its usefulness, the Fourier transform has been adapted for use on the personal computer. In other cases, it can identify the regular contributions to a fluctuating signal, thereby helping to make sense of observations in astronomy, medicine and chemistry. In some cases, the Fourier transform can provide a means of solving unwieldy equations that describe dynamic responses to electricity, heat or light. The Fourier transform has become a powerful analytical tool in diverse fields of science. Figure 1 illustrates this time to frequency domain conversion concept. Plotting the amplitude of each sinusoidal term versus its frequency creates a power spectrum, which is the response of the original waveform in the frequency domain.
FOURIER TRANSFORM SERIES
This process, in effect, converts a waveform in the time domain that is difficult to describe mathematically into a more manageable series of sinusoidal functions that when added together, exactly reproduce the original waveform. The Fourier transform accomplishes this by breaking down the original time-based waveform into a series of sinusoidal terms, each with a unique magnitude, frequency, and phase. Simply stated, the Fourier transform converts waveform data in the time domain into the frequency domain. The Fourier transform is the mathematical tool used to make this conversion. The brain then turns this information into perceived sound.Ī similar conversion can be done using mathematical methods on the same sound waves or virtually any other fluctuating signal that varies with respect to time.
The ear formulates a transform by converting sound-the waves of pressure traveling over time and through the atmosphere-into a spectrum, a description of the sound as a series of volumes at distinct pitches. The human ear automatically and involuntarily performs a calculation that takes the intellect years of mathematical education to accomplish. To calculate an FFT (Fast Fourier Transform), just listen. WinDaq addons provides live FFT and many more features
FOURIER TRANSFORM FOR FREE
Signup on YourEngineer and get 1000 Engicoins instantly.Order a DI-188 WinDaq starter kit for $49 and get WinDaq addon for FREE to study FFT ! The Fourier Transform can be used to study signals in a variety of applications, such as image and audio processing, medical imaging, and communications engineering. The Fou rier Transform can also be used to reconstruct a signal from its frequency components. This information can be used to filter out unwanted frequencies or to enhance certain frequencies. The transform produces a frequency spectrum, which can be used to identify the frequencies present in a signal. The Fou rier Transform can be used to analyze the frequency content of a signal. In practice, the Fou rier Transform is usually computed using the Fast Fou rier Transform ( FF T ) algorithm, which is a much faster and more efficient method than the traditional integral approach. This process is known as conv olution and it can be used to obtain the Fou rier Transform of a signal. The Fou rier Transform is an integral transform, meaning that the transform of a signal is computed by integrating the signal over time.


 0 kommentar(er)
0 kommentar(er)
